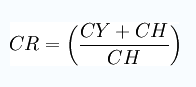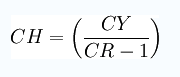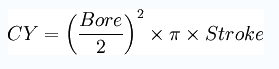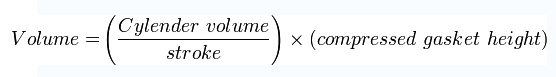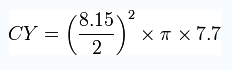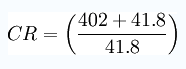Calculating Compression Ratios
Many changes to the components or state of some parts in an engine will affect the static compression ratio. This with some known figures can be calculated and used as a tool in choosing parts for the assembly of an engine.
Formula used
To work out what the initial C.R (compression ratio) is or how it is affected by engine setup changes the following formula are used:
which can also be transposed into this formula:
Abbreviations used
Note: All volumes expressed in CC (Cubic centimeters)
CR: The static compression ratio, expressed as a ratio of the uncompressed volume to the compressed volume, (Ex: 11.5:1)
CH: The chamber volume, which is worked out as follows- (combustion chamber volume) + (displaced volume of head gasket) + (spark plug volume) + or – (piston displacement, volume added if the piston is dished or volume deducted if the piston is domed).
CY: The cylinder volume, this is the volume displaced by the cylinder. Dividing the engines known displacement by the amount of cylinders is usually inaccurate (ex: 1600c/4), this is because the quoted displacement is always a rounded off figure (ex: a 4age is 1587cc, a “1.6L engine”. The better way to work out the cylinder is shown in the next section.
Working out the cylinder volume
The formula for working out the volume is shown below:
Using the following:
π: Pi, which is 3.141 or (22/7)
Bore: The diameter of the bore in Cm.
Stroke: The length of the stroke in cm.
A practical example:
A 4age 20V Silvertop engine has a known Bore and stroke of (81×77) in mm and a compression ratio of 10.5:1
So converting the bore and stroke from mm to cm (x/10) the Bore is 8.1 and the stroke is 7.7
Firstly the cylinder volume is worked out using the formula:
By putting in our bore and stroke we end up with the following:
Working it out we get 396.7, which we for simplicity will round up to 397CC.
Now that we know the cylinder volume we go to the original formula:
By substituting the known numbers we end up with the following:
This works out to be 397/9.5 this equals 41.8cc, this is the total volume in the chamber.
Working out the change in compression ratio from a change in the chamber size
If something reduces the chamber size such as a thinner head gasket, shaved head, a different head altogether, etc.. then the compression ratio will rise.
To work out the change the original figures are required for the engine such as the cylinder size, bore, stroke, original C.R and original chamber size. This can be worked out by using the examples shown earlier.
Then the change in displacement needs to be worked out. If for example a thinner head gasket is used then the following need to be calculated: the original volume displaced by the compressed gasket, the new gasket compressed volume and then the difference. Or the heigh of one is deduced from the other and then the volume calculated from the final height. This final figure will then need to be deducted from the original chamber size and the new C.R calculated accordingly.
An example:
Again using the previously mentioned 4age 20V Silvertop engine:
CY = 397CC CH = 41.8CC C.R = 10.5:1 (originally)
Now the original gasket is reported to have a 1.15mm compressed thickness and a 0.8 metal TRD gasket is used the compressed height is meant to be as the name implies: 0.8mm. So deducting one from the other the change in height is -0.35mm or 0.035Cm
To work out the displacement easily of any given gasket just use the following formula:
Because we only want to work out the change we use the figure of 0.35mm
( 397CC / 77mm ) x 0.35mm = 1.8CC
So therefore using the reported figures the change in chamber volume when changing to this gasket would be -1.8CC.
The next step is to work out the change to the total chamber size and then finally the overall new compression ratio.
The original chamber size was 41.8CC as worked out earlier, and the change with the gasket was -1.8CC, so with some simple maths, the new chamber size will be 40cc.
Transposing this back into our first formula:
so therefore the final compression ratio will be 10.925:1
To work out the change resulting from shaving a cylinder head is it much the same as above, with the exception that the amount of material shaved off is used directly in place of the change of gasket height, the rest of the steps are identical.
Working out the change in compression ratio from a change in the cylinder size through bore or stroke
If something increases the cylinder size such as an overbore to suit a larger diameter piston, or if the stroke is increased then the compression ratio will also rise.
To work out the change the original figures are required for the engine such as the cylinder size, bore, stroke, original C.R and original chamber size. This can be worked out by using the examples shown earlier.
Then the change in volume needs to be worked out. If a cylinder is machined to have a 1mm over-bore then the new cylinder size needs to be worked out and then the C.R re-calculated.
For example if the earlier quoted 4age 20V Silvertop is machined to have a 0.5mm overbore then the new cylinder needs to be worked out. This is easily done by using the earlier formula:
By substituting the previously known numbers but with a new bore of 8.15(cm) we end up with the following:
and therefore the new cylinder volume would be 402, now by substituting the new cylinder size back into the first formula:
So therefore the new compression ratio is 10.62:1
Working out the change of C.R from an increase in stroke is virtually identical as what is shown above with an increase in bore. The difference being the figure for the stroke is changed when working out the cylinder volume and then it is substituted back into the first formula.
Working out the change in compression ratio from a change in the cylinder head to a different model
If the cylinder head is being changed to a model that has a different combustion chamber volume then the process of working out the resulting compression ratio is significantly harder. To do this the volume of both cylinder heads is needed (not the total chamber volume). The difference then needs to be deducted/added to the total chamber volume and the new C.R worked out accordingly.
For example if the earlier quoted 4age 20V Silvertop is to have a later model blacktop fitted:
The Silvertop head is 35cc (measured by Arias pistons)
The blacktop head is 37.8cc (measured by myself)
37.8 – 35 = 2.8CC
So therefore the head chamber will end up being 2.8cc larger, this can be directly added to the total chamber size:
41.8 + 2.8CC = 43.6CC
So the new total chamber size (CH) will be 43.6CC
now by substituting the new total chamber size back into the first formula:
So therefore the new C.R will be 10.11:1